Abstract

This paper intends to analyze the debt threshold for Pakistan over a period of 1976-2020 within the framework of the ARDL bounds testing approach. ADF and PP tests of stationarity confirmed that the extracted data set was stationarity either at level or at first difference. The F-bound test validated the presence of long-run cointegration. This paper finds that the curve for the bivariate quadratic function is strictly concave and that the debt inflexion point is 48.42 percent of the gross domestic product. It implies that the marginal effect of debt turns negative beyond this level of debt. Findings suggest that government should ensure to acquire external debt upto the debt-inflexion point that would enhance the economic growth. Government should also work on fiscal consolidation and substituting the required burden of debt with alternate sources of revenue like FDI, grants, and international trade.

Key Words

Debt-threshold, Debt-Laffer Curve, ARDL, Pakistan

Introduction

The global financial crises of 2007-2008 and debt-crises in different eras around the globe were the two fundamental causes of building the interest of researchers on investigating the debt-growth nexus, especially in developing economies who were engaged in borrowing primarily for meeting with their financial obligations and carrying their developmental schemes (Minhaj-ud-Din, Azam & Tariq, 2021). Different routes and channels of transmission have been identified by many researchers. The neoclassical economists  are of the view that an increase in cost of debt is normally paid through bringing increase in taxes which affects the stock of capital negatively and, hence, the growth process of the concerned economy deteriorates (Diamond, 1965). In contrast, Keynesian economists claim that the impact of indebtedness is effective as a rise in debt induces public spending, which poster a positive multiplier effect on the economy (Salmon, 2021). The "conventional view" also believes on its short-run impact on the economy (Heimberger, 2021b). While investigating the notions of neoclassical economists, the routs through which foreign debt are presumed to dampen the economy are, first, debt-overhang effect of the external debt caused by high distortionary taxes that are required for servicing the future debt liabilities (Krugman, 1988; Sach & Williamson, 1985; Borenztein, 1990; Ajayi, 2000; Elbadawi et al., 1997; Akram, 2014; Fandamu & Phiri, 2017; Minhaj-ud-Din, Azam & Tariq, 2020) and, second, debt crowding-out caused by the competition for funds in the capital market where private investment is crowded out by the government borrowing due to involvement of high-interest rate (Chowdhury, 1994; Claessens & Diwan, 1990; Presbitero, 2006; Akram, 2014, Fabdamu & Phiri, 2017; Minhaj-ud-Din, Azam & Tariq, 2020). Eventually, the investment and economic growth of such economies are disrupted, and they are trapped in a vicious circle of debt. Moreover, the adverse effect of higher debt accumulation can be more precarious in the long run if the higher debt is expected to be the cause of higher inflation and financial subjugation (Cochrane, 2011).

Debt Profile of Pakistan

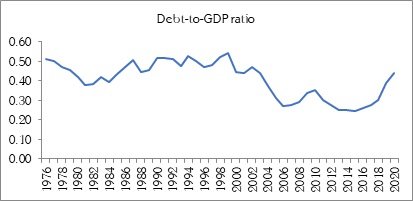
Figure 2 and Figure 3 illustrate the foreign debt profile, in terms of quantification and comparative analysis with DGP, for the period under analysis. Reasons for choosing external debt, rather than domestic debt, as a research topic, are: first, the uncontrollable impact of foreign currency on interest and exchange rates; second, swallowing a major portion of government revenues earned in foreign currency. Third, destructing the roots of the domestic economy through intervention in its macroeconomic environment and, fourth, devaluation of local currency that causes growth in the value of external financial obligations.

Figure 2

Debt-Profile of Pakistan (1976-2020)



Figure 3

Debt-to-GDP ratio of Pakistan (1976-2020)



Keeping in view the importance of external debt in shaping the growth process, we find several groups of researchers that have rigorously investigated the debt-growth nexus; however, we find only a few studies that have investigated the issue of debt-threshold for Pakistan. So keeping in view the scarcity of literature on the estimation of debt ceiling point, this paper is primarily focused on quantifying the debt inflexion point for Pakistan, beyond which a slight increase in acquisition of foreign debt is considered a sole cause of slack economic growth.

Literature Survey

Researchers have used extensive examination techniques for the purpose of investigating the debt threshold empirically. However, controversies in their results is still alive in the existing literature. The majority of these researchers believe that debt-to-GDP ratio had a strong negative association with the economic growth beyond the debt inflexion point. They believe that the acquisition of debt leads to dampen the growth process above the debt-inflexion point. Few researchers have partly reported at best that this association is pathetic and fragile. Similarly, several others have pointed the lack of evidence for the existence of a universal debt-threshold.

Keeping in view the theoretical and empirical perspectives of literature related to debt-driven slack economic growth, controversies in the conclusions of these literatures have sparked a major debate over this issue. A brief introduction towards the most important segments of the theoretical and empirical literature is focused in the following section.   

Theoretical Literature

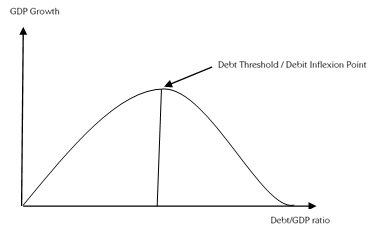
Debt limit/ceiling is the maximum amount of debt that can be owned by a government for the purpose of financing the twin deficits, completion of developmental projects, and bringing growth and prosperity in the macroeconomic indicators of an economy (Reinhart & Rogoff, 2010; Ogunmuyiwa, 2011; Nwankwo, 2014; Omotosho et al., 2016). It is that rank of government debt where the marginal effect of such debts turns negative (Minhaj-ud-Din, Azam & Tariq, 2021). Researchers have shown a soft corner for the acquisition of foreign debt as far as the acquired fund is, first, below the debt-threshold and, second, utilized in productive channels (Nguyen et al., 2003; Cordella et al., 20010; Greenidge et al., 2012; Van, 2018; Zaghdoudi, 2019; Lio & Lyu, 2021; Minhaj-ud-Din, Azam & Tariq, 2021). They also suggest that, at need time, the gap between debt and GDP (i.e. debt-to-GDP ratio) must be minimal; otherwise, higher public debt will lead to slumped economic activities in the form of lower economic growth, higher taxation, higher inflation, income inequality, and to intergenerational inequality in the long run (Cochrane, 2011). Supporters of this conception believe that growth is inversely affected by debt in the long run, where an increase in government debt is considered beneficial for growth and prosperity below the debt inflection point, but if the public debt exceeds the debt threshold, then a slight increase in debt will create disincentives for the economy and will slip it into deep debt-trap (Mupunga, 2015; Van, 2018; Khanfir, 2019; Ndoricimpa, 2020; Minhaj-ud-Din, Azam & Tariq, 2021). The non-linear relations of debt-to-GDP growth can be expressed with the help of the following Debt-Laffer curve (Figure 1).

Figure 1

Debt-Laffer-Curve



Empirical Literature

The most influential paper on this topic is the article written by Reinhart and Rogoff in 2010, where they tried to emphasize on the significance of debt during the period of debt-crises. In this paper, data sample was derived from 44 countries which were classified into four clusters; economies with lower-debt burden (i.e. debt-to-GDP < 30%), medium-low debt burden (i.e. 30% < debt-to-GDP < 60%), medium-high debt burden (i.e. 60% < debt-to-GDP < 90%), and high-debt burden (i.e. debt-to-GDP > 90%). Total observations in this study were 3700, spanning over a period of about two hundred years (1790-2009). They found that countries with higher growth tend to observe lower growth rates. Kumar and Woo (2010) used the GMM estimation technique for analyzing this inverse relationship and noted that growth in the debt-to-GDP ratio is connected with a slack economic growth. On average, 10 percentage point increase in this ratio was found to drag down the annual growth of real GDP by 0.25 percentage points. Cordella et al. (2010) concluded that the negative collision of foreign indebtedness is unyielding for countries where the level of debt-threshold ranges between 15 to 30 percent of GDP. However, this effect was found extraneous for those having a debt threshold of 70 to 80%. For Greenidge et al. (2012) this ratio was 55 % of the gross domestic product.

Mupunga and Roux (2015) and Chudik et al. (2015) analyzed the Debt-Laffer theory but failed to detect a universal debt threshold for the economies under analysis. Omotosho et al. (2016) and Cai (2017) also examined this non-linear relationship and portrayed that the involvement of a country in debt over and above the debt threshold will always lead to a debt overhang effect. Van (2018) and Tran (2018) triggered on investigating the debt-inflexion point and confirmed that this relationship yields to inverted-U shape curve, quite resembled to debt-Laffer curve. They concluded that debt flight over the level of debt-threshold will always lead to dampening the economic growth. Khanfir (2019) and Zaghoudi (2019) stressed on fiscal consolidation and dragging the debt burden below the debt threshold towards stimulating economic growth. Ndoricimpa (2020) and Bhatta and Mishra (2020) focused on accelerating the economic growth and reducing the negative impact of debt which was found drastic in debt-abundant countries as compared to debt-moderate economies.

With regards to the application of this theory in Pakistan, we have come across only a few papers that have examined the theories and concepts of this inverse relationship and have concluded that this relationship exists in the form of an inverted-U shape curve. This paper is an addition to the available literature related to the analysis of debt thresholds that focuses on policy perspectives as well.   

Data and Data Source

The data source for all variables of the model is World Development Indicators (2021), except the exchange rate which is extracted from Economic Research Division (2021). The time period ranges from 1976 to 2020.




Estimation Procedure

ARDL bound testing approach is used as estimation technique. Variable “ed2” is inserted with the intention to calculate the debt-inflexion point from the following ARDL model:

?g_t= ?_0+?_(i=1)^n???_1 ?g_(t-1) ?+?_(i=0)^n???_2 ??ed?_(t-1) ?+ ?_(i=0)^n???_3 ???ed?^2?_(t-1) ?+?_(i=0)^n???_4 ??dsp?_(t-1) ?+  ?_(i=0)^n???_5 ?? ?fdi?_(t-1)+?_(i=0)^n???_6 ??er?_(t-1) ?+?_(i=0)^n???_7 ?? ?gfcf?_(t-1)+?_(i=0)^n???_8 ?? ?hk?_(t-1)+?_(i=0)^n???_9 ??inf?_(t-1) ?+?_(i=0)^n???_10 ??lf?_(t-1) ?+?_(i=0)^n???_11 ?? ?fer?_(t-1)+?_t………….. (1)

After the regression, debt-threshold can be obtained by substituting the coefficients of "ed” and “ed2" in equation 2. The issue of relative maxima and minima can be encountered through the application of the first-order and second-order derivatives to this model with respect to the external debt (equation 3).

Debt inflexion point={((coefficent of ed))/((2*coefficient of ?ed?^2 ) )}……………(2)

The Analysis of Maxima and Minima

After regressing equation 1, but the values of resulted coefficients back in this equation for the

 purpose of resolving the issue of relative maxima or minima. In this regard, we have to take the derivative of this equation with respect to “ed”. Mathematically:



The necessary condition for relative maxima (concavity) is that f ? (ED) = 0, and its sufficient condition is that f ?? (ED) < 0. With this formulation, one can easily determine whether the bivariate quadratic function is strictly convex (U-shape) or strictly concave (inverted-U shape). A debt inflexion point can also be calculated by putting the resulted value of coefficients in the debt-threshold model (equation 3) and taking its derivative with respect to “ed”.

Regression Results

Before applying the ARDL model, it stands radical to resolve the issue of stationarity by applying the two famous estimation techniques, ADF and PP tests of stationarity. The resulted values of all variables of the model were found stationary either at I₍₀₎ or at I₍₁₎.

F-Bound Test

Outcomes of the F-bound test, depicted in Table 1, specify, first, the presence of long-run cointegration and, second, all variables of the model are the factors that determine the growth in the long run.




Table 1. F-Bound Test

Cal F-Value	6.726
	LB	UB
10 percent	1.88	2.99
5 percent	2.14	3.3
2.5 percent	2.37	3.6
1 percent	2.65	3.97

 




Table 1 indicates the presence of long run cointegration as the calculated F-value > LB & UB at all levels of significance. So we have to move further towards the estimates of ARDL model.

Results of the ARDL Model

As we know that for estimating the threshold level, it is mandatory that, first, the coefficient of “ed” variable should be > 0, and, second, the estimate of “ed²" should be < 0. In other words, the slope of demand function should increase with a decreasing rate while reaching to the maximum and then should turn negative for showing a debt-threshold. Table 2 enlists the results of regression analysis and prove that; first, the resulted value of “ed > 0” and, second, “ed² < 0”. Moreover, both these variables are significant at 5% and 1% percent, respectively.




 

Table 2. Analysis of the Debt-threshold

variable	coefficient	t-statistic	p-value
ed(t)	0.93492*	0.31054	0.0094
ed2(t)	-0.00971*	-3.03923	0.0088
dsp(t)	-0.16214**	-2.78620	0.0146
fdi(t)	1.01032**	2.39390	0.0302
er(t)	0.10732**	2.60830	0.0206
gfcf(t)	0.69110*	4.57291	0.0004
hk(t)	0.15752**	1.97542	0.0483
inf(t)	-0.12254**	-2.08700	0.0357
lf(t)	1.93777*	4.79112	0.0003
Fer(t)	1.26481*	3.97886	0.0014
c	-75.54644	-2.50924	0.0250

Note: ^* and ^** tells us about the level of significance

 




Table 2 also supports our stance presented in the theoretical literature portion of this study that acquisition of foreign debt is beneficiary only up to the debt threshold point, and slight growth in debt accumulation will beyond this point have a significant effect on the growth process of Pakistan. The co-existence of overhang and crowding-out effects are also associated with these values. The debt-threshold point can also be observed from the negative signs of the resulted values associated with variables “ed²” and “DSP". These findings are supportive of the conclusion of our previous study on this topic (i.e. Minhaj-ud-Din, Azam & Tariq, 2020). Discussion on the findings of remaining variables can also be made here easily, but since our basic objective is to calculate the debt threshold, that's why we are moving further towards the analysis of the debt threshold.

Analysis of the Relative Maxima and Minima

Mathematically, it is easy to sort out the relative maxima or minima. By doing so, we have to take the resulting values of "ed” and “ed2" from Table 2 and put them in equation 3. After doing so, apply the derivatives and make the resulted equation isequal to zero. By doing so, we will obtain the following bivariate quadratic function. Mathematically:

f ? (ed)=d/dG(0.9349 *ed)-d/dG(0.0097 ?ed?^2)

f ? (ed)=0.9349-2*0.0097 ED = 0

f ? (ed)=0.9349-0.0194 ED = 0 ……… (4)

While moving further, we have to apply the necessary condition of relative maxima/minima. Theoretically, these conditions are:

Necessary Condition: f ? (ed)  = 0

Sufficient Condition: f ?? (ed)<0

After applying the necessary condition equating equation 4 to zero, now it is easy to verify that f ? (ed)  > 0 for all values of “ed” < 48.42, and f ? (ed)  < 0 for all values of “ed” > 48.42. To check whether the derived estimate represents the optimum debt threshold (inverted-U shape curve) or not (U-shape curve), we have to apply the second-order condition (sufficient condition) to equation 4. Mathematically:

f ?? (ed)=-2*0.0108

f ?? (ed)=-0.0216 < 0

Since the resulted value of the second-order condition is negative, hence proved that this value represents the debt-threshold and that this point is the relative maxima (i.e., optimum debt-threshold) and the graph for this quadratic function will be an inverted U-shape curve that can be represented with the help of Figure 4.

Figure 4





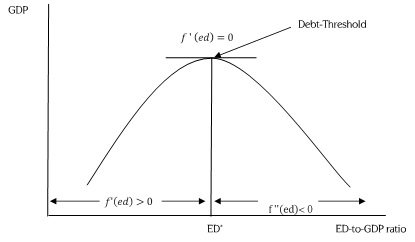
Figure 4

Figure 4 indicates that to the left of the optimum rate of external debt (i.e., ED*), the magnitude of external debt increases with a decreasing rate while reaching to zero at boom where the slope of the debt-curve is zero (i.e.,f ? (ed)  = 0, necessary condition of a maxima). After crossing this optimal point, the slope of debt-curve s negative (i.e.f ?? (ed)  < 0), which is the sufficient condition of a maxima. This figure also illustrates that, first, the debt curve for this bivariate quadratic function (i.e., equation 4) is strictly concave or inverted U-shape curve which shows an inverse relationship of growth with debt and, second, that the resulted value (i.e., 48.42 percent of GDP) is an optimum value of debt-threshold beyond which a slight increase in debt acquisition will disrupt the whole growth process and will lead to foster adverse effects on the economy. Further, these estimates are similar to the conclusion made by Nguyen et al.(2003), Mupunga and Roux (2015), Baharumshah et al. (2016), Tran (2018), Khanfir (2019), Zaghdoudi (2019), and Bhatta and Mishra (2020). These results also highlight the need of special policy intervention from the central government for keeping the magnitude of debt below the debt threshold. This issue is of serious concern that needs to be resolved on a priority basis, otherwise, the attitude of the government regarding taking "loan on loan" and "loan for the loan" will not only paralyze the federal government but will also pose a risk of bankruptcy in the future.

Conclusion and Policy Recommendations

As we know that external borrowing is not avoidable completely therefore fixing the debt-ceiling point is mandatory for debt accumulation, policy guidelines, and adequate policy implications. This study was aimed to cope with this issue by using the ARDL bound test to cointegration. For this purpose, a detailed estimation process has been deployed in this paper. The data period was ranging from 1976 to 2020 and its sources were WDI (2021) and Economic research division (2021). This study finds that the debt threshold in Pakistan is 48.42% of our GDP, and an increase in the accumulation of debt beyond this level will lead to dampening economic growth. For this purpose, the government should focus on fiscal consolidation and should focus on substituting the required fund with other sources of revenue like FDI, grants and international trade. Government should also ensure to acquire external debt up to the debt-inflexion point that would enhance the economic growth. If the issue of taking a loan on loan, and even loan for the loan, was not handled properly, then economists have already expressed their reservations that this attitude of the federal government will eventually paralyze him, and he will have even no money for payment of salaries to the government servants in the long run. Eventually, it will not only pose a risk of bankruptcy but will also threaten our integrity and security in the long run. 

References

• Ajayi, S. (2000). Macroeconomic approach to external debt: The case of Nigeria. External debt and capital flight in sub-Saharan Africa, 11-56.
• Akram, N. (2014). Empirical examination of debt and growth nexus in South Asian countries. Asia-Pacific Development Journal, 20(2), 29-52.
• Baharumshah, A. Z., Soon, S. V., & Lau, E. (2017). Fiscal Sustainability in an Emerging Market Economy: When does Public Debt Turn Bad? Journal of Policy Modeling 39(1), 99- 113.
• Bhatta, G. P., & Mishra, A. (2020). Estimating Optimum Growth-Maximizing Public Debt Threshold for Nepal. NRB Economic Review, 32(2), 1-28
• Borensztein, E. (1990). Debt overhang, debt reduction and investment: The case of the Philippines. IMF Working Paper No. WP/90/77.
• Cai, Y. (2017). Nonlinear Analysis of Economic Growth, Public Debt and Policy Tools. Asian Economic and Financial Review 7(1), 99-108.
• Chowdhury, K. (1994). A structural analysis of external debt and economic growth: some evidence from selected countries in Asia and the Pacific. Applied Economics, 26(12), 1121-1131.
• Chudik, A., Mohaddes, K., Pesaran, M. H., & Raissi, M. (2017). Is there a debt-threshold effect on output growth? Review of Economics and Statistics, 99(1), 135-150.
• Claessens, S., & Diwan, I. (1990). Investment incentives: new money, debt relief, and the critical role of conditionality in the debt crisis. The World Bank Economic Review, 4(1), 21-41.
• Cochrane, J. (2011): Understanding policy in the Great Recession: some unpleasant fiscal arithmetic, European Economic Review, 55(1), 2-30.
• Cordella, T., Ricci, L. A., & Ruiz-Arranz, M. (2010). Debt overhang or debt irrelevance? IMF Staff Papers, 57(1), 1-24.
• Diamond, P. A. (1965). National Debt in a Neoclassical Growth Model. American Economic Review 55 (5): 1126-50.
• Economic Research Division (2021). Government of Pakistan, Ministry of Economic Affairs and Statistics, Islamabad.
• Elbadawi, I., Ndulu, B. J., & Ndung'u, N. (1997). Debt overhang and economic growth in Sub-Saharan Africa. External finance for low-income countries, 49-76.
• Fandamu, L. D. S. K. H., & Phiri, C. (2017). The Impact of External Debt on Zambia's Economic Growth: An ARDL Approach. Journal of Economics and Sustainable Development, 8(8), 55-68.
• Greenidge, K., Craigwell, V., Thomas, C., & Drakes, L. (2012). Threshold Effects of Sovereign Debt: Evidence from the Caribbean. IMF Working Paper 157, 1-22.
• Heimberger, P. (2021b): Does employment protection affect unemployment? A meta- analysis, Oxford Economic Papers, 73(3), 982-1007.
• Khanfir, W. (2019). Threshold effect of public debt on economic growth: An empirical investigation for selected North African countries. Economic Alternatives, 3, 429- 436.
• Krugman, P. R. (1988). Market-based debt- reduction schemes. Journal of Development Economics, 29, 253-268.